Geometriphylogenetics
« previous post | next post »
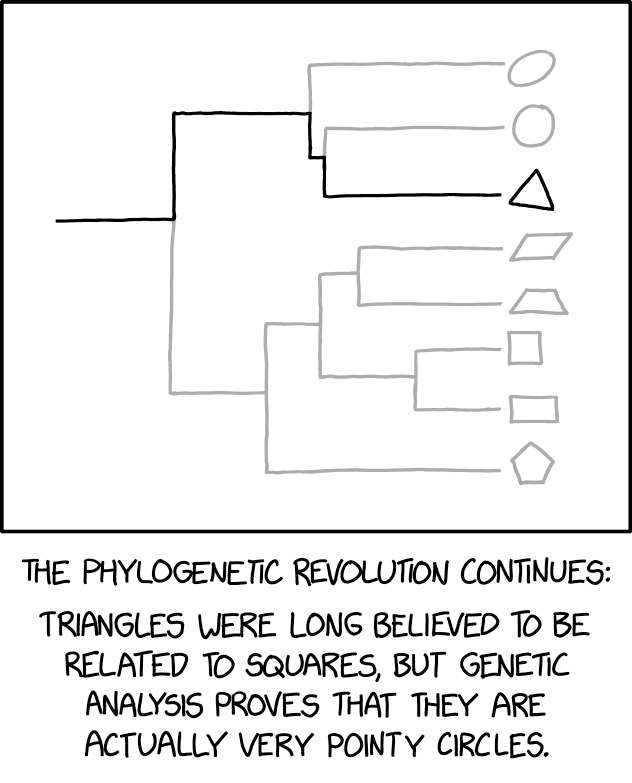
Mouseover title: "There's a maximum likelihood that I'm doing phylogenetics wrong."
It's not that Randall is "doing phylogenetics wrong", but rather than he's applying it to an inappropriate problem.
The OED's etymology for phylogeny is
< German Phylogenie (E. Haeckel Gen. Morphol. der Organismen (1866) I. iii. 57) < Phylum phylum n. + ‑o‑ ‑o‑ connective + ‑genie ‑geny comb. form.
The Greek source for phylum is φῦλον, glossed as "race, tribe, or class" and various related concepts. And the OED's earliest English citation for phylum is from T.H. Huxley, Proceedings of Zoological Society, in 1868:
The relations of the different groups should be capable of representation by a genealogical tree, or phylum as Haeckel calls it in his remarkable ‘Generelle Morphologie’.
What all of these have in common is the idea of a tree-structured taxonomy. In the case of animals, this is pretty much guaranteed by the nature of generation-to-generation descent — though the situation for bacteria is different, since different lineages can exchange genetic material across well-separated geneological branches.
Of course, viruses (and human biologists) can do a version of the same thing. And there's a long history of debate about wave models versus tree models of linguistic evolution.
Anyhow, there's no basis — in math or psychology — for a single well-defined tree model for geometric figures. (And of course "genetic analysis" is not coming to the rescue.)
There are plenty of kinds of cultural evolution where it's clear that the history is not tree-structured:
"The evolution of the cocktail", 7/20/2010
"Folktale phylogeny". 1/25/2016
JPL said,
November 12, 2024 @ 4:29 pm
What about Felix Klein's Erlangen Program? And how might that be related to the reality described by phylogenetics in biology (or the forms used to describe it, for that matter)?
Seth said,
November 12, 2024 @ 6:25 pm
It's not a "tree" model, but the joke is obviously playing off the organizational sequence of elementary geometry which runs:
triangle -> square -> pentagon -> hexagon -> … -> circle
i.e. a circle is the "evolutionary result" of figures "evolving" by increasing their number of sides but shortening them to roundness. Thus squares are evolved triangles. But the joke is that triangles are circles (no sides) which in fact evolved a few short and pointy sides.
Brett said,
November 12, 2024 @ 8:42 pm
@Seth: In Flatland, triangles evolve to be closer to equilateral over the generations.
Then their progeny gain a side with each of the next several generations. Polygons with more sides have higher social status, and as the number of sides increases, so eventually does the rate at which they are added, so that the caste of high priests are indistinguishable from circles.
번하드 said,
November 14, 2024 @ 2:00 pm
Ah, nice to see a mention of Flatland. I read that book at highschool, at the recommendation of my math teacher.
A few months ago I stumbled across an animated movie along similar lines:
https://youtu.be/avMX-Zft7K4
thunkii said,
November 18, 2024 @ 9:05 pm
I remember an exhibit of bird at the Field Museum of Chicago which was meant to be arranged phylogenetically. But later advancements proved their layout to be completely wrong. For instance, loons and grebes were thought to be very closely related water birds (and placed close to each other in the exhibit), but grebes are actually in their own clade together with flamingos. Naturally, the museum tried to hastily resolve this with explanatory stickers all over the glass.
Michael Watts said,
November 19, 2024 @ 5:12 am
Why wouldn't the resolution be to just rearrange the exhibit?